'Maths is often way ahead of practical applications'
A secret code that we currently use to send e-mails securely is based on the maths of a century ago. The geometrical surfaces that Dino Festi studied during his PhD research will perhaps be used in future codes or new physics. PhD defence 5 July.
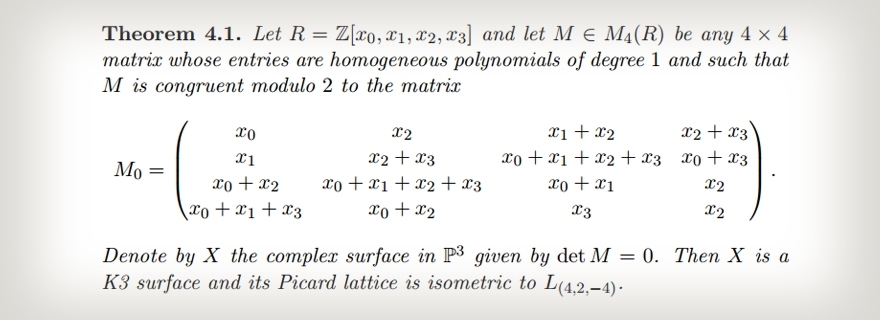
Many mathematical formulae can be imagined as complex folded surfaces in space. PhD candidate Dino Festi studied K3 surfaces. Festi: 'K3 surfaces are at the interface between surfaces that we are able to understand and surfaces about which we understand nothing.' This is what led him to choose this as the subject for his research.
'Understanding' surfaces
'Understanding' surfaces means that solutions will be found to the mathematical equations with which the surfaces correspond. Calculating equations is algebra; studying surfaces is geometry. Festi's field brings the two domains together: algebraic geometry.
Equations in ancient Greece
The origin of Festi's field of research is ages old. Most people are familiar with Pythagoras's theorem: a2 + b2 = c2 . It is a theory formulated in ancient times by Greek mathematicians, and it indicates the relationship between the sides of a right-angled triangle with the lengths a, b and c. If you are free to choose a and b, you can easily calculate c. But if you set the extra condition that a, b and c have to be whole numbers, it becomes a lot more difficult.
Rational numbers
Dino Festi's research is distantly related to this. He focuses on a family of highly complex equations, and tries to find solutions that have to be rational numbers. A rational number is a fraction of whole integers a and b, in other words a/b. Then, even what appear at first sight to be quite simple equations can be full of enigmas. An equation from this family corresponds with a curved and folded surface in three-dimensional space. Because Festi only looks at rational solutions, it can be imagined that this space is filled with a grid of separate points with rational coordinates. Festi wants to know exactly which of these grid points are found on the surface in question.
Zero or infinite
There are well-known examples of K3 surfaces on which there are no or an infinite number of rational points. But is has not yet been proven that there are no surfaces with a finite number of rational points. Festi's most important result relates to these K3 surfaces. 'I have worked out the characteristics of the curves that traverse these surfaces,' he explains. The meridians of a globe are examples of such curves, but on a rounded surface. Anyone who is able to describe these curves in mathematical terms also has a good understanding of the surface as a whole.
Research across the globe
According to Festi, these kinds of questions are being studied by colleagues across the globe. If anyone eventually does fathom out a mathematical object, often a tool can be made that will be useful in other sciences. There is, for example, a link between K3 surfaces and string theory in physics: the much-dreamed-of 'theory of everything'. For Festi himself, the motivation is more aesthetic: 'They are beautiful objects. From October I will be doing postdoctoral research in Mainz, on this same subject. It's something I can study for years to come.'
